Equations of conic sections
Here we will have a look at three different conic sections:
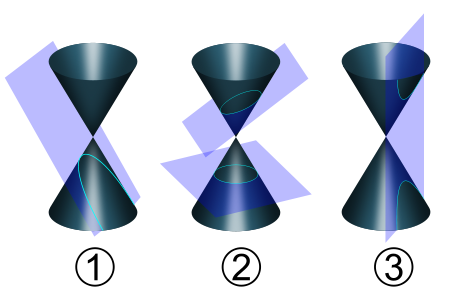
1. Parabola
The parabola is a conic section, the intersection of a right circular conical surface and a plane parallel to a generating straight line of that surface. The equation for a parabola is
$$y=a(x-b)^{2}+c\; or\; x=a(y-b)^{2}+c$$
2. Circles and ellipses
The equation of a circle with center at (a,b) and radius r units is
$$(x-a)^{2}+(y-b)^{2}=r^{2}$$
An ellipse is the figure consisting of all points in the plane whose coordinates satisfy the equation
$$\frac{x^{2}}{a^{2}}+\frac{y^{2}}{b^{2}}=1$$
If the ellipse has its center at (m,n) the equation could be written as
$$\frac{(x-m)^{2}}{a^{2}}+\frac{(y-n)^{2}}{b^{2}}=1$$
3. Hyperbolas
A hyperbola is a curve, specifically a smooth curve that lies in a plane, which can be defined either by its geometric properties or by the kinds of equations for which it is the solution set. A hyperbola has two pieces, called connected components or branches, which are mirror images of each other and resembling two infinite bows.
The equation of a hyperbola with a center at (m,n) is
$$\frac{(x-m)^{2}}{a^{2}}-\frac{(y-n)^{2}}{b^{2}}=1$$
Video lesson
Write the given equation on the standard equation form for ellipses
